The Doppler broadening of spectral lines
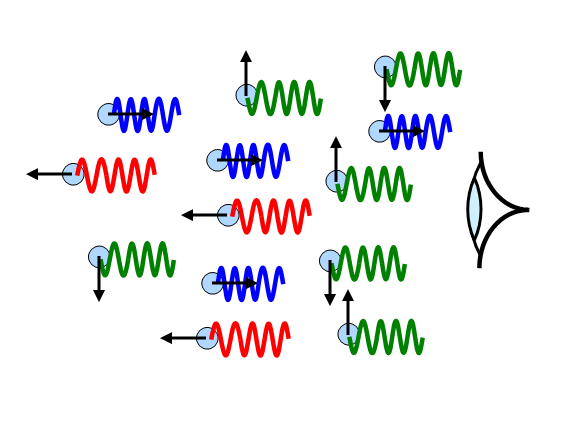
In a plasma (a gas at very high
temperature) the atoms move at very high velocities. The light emitted by the atoms will therefore
be Doppler-shifted. Since some atoms will be moving towards an observer and others away from
them, the result will be that each line in the spectrum will be broadened.
The width Dl of a given line of wavelength l can be
shown to be:
Dl = 2vl/c
where v is the r.m.s velocity of the atoms. From the gas laws,
however, we have that
v = [3RT/M]
1/2 where M is the molar mass of
the gas, R the gas constant and T the absolute temperature of the gas.
Combining these two
equations thus gives us a means of finding the temperature of the gas from the Doppler
broadening of the spectral lines:
Temperature (T) = [Dl2c2M]/12l2R
Example problem
Calculate the broadening of a line of mean wavelength 656 nm in a hydrogen plasma at 106 K.
(Take the molar mass of hydrogen in a plasma to be 0.001 kg.) (Gas constant R = 8.13 Jmol-1)
Dl = [12l2RT/c2M]1/2 = [4.29x1013/9x1013]1/2 = 0.69 nm
A VERSION IN WORD IS AVAILABLE ON THE SCHOOLPHYSICS USB


